Problem 1
Consider an unbiased p-n junction.
(a) Draw the band diagram (conduction band, valence band, Fermi energy).
(b) As the pn-junction is heated up, the Fermi energy moves with respect to the band edges $E_c$ and $E_v$. How does it move? What happens to the built-in voltage as the p-n junction warms up?
(c) The p-n junction is forward biased with a voltage $V$. What is the maximum concentration of holes on the n-side and the minimum concentration of holes on the n-side? Give your answers in terms of the donor concentration \(N_d\), the acceptor concentration \(N_a\), and $n_i$.
(d) Light falls on the p-n junction. Does the current flow towards p or towards n?
Solution
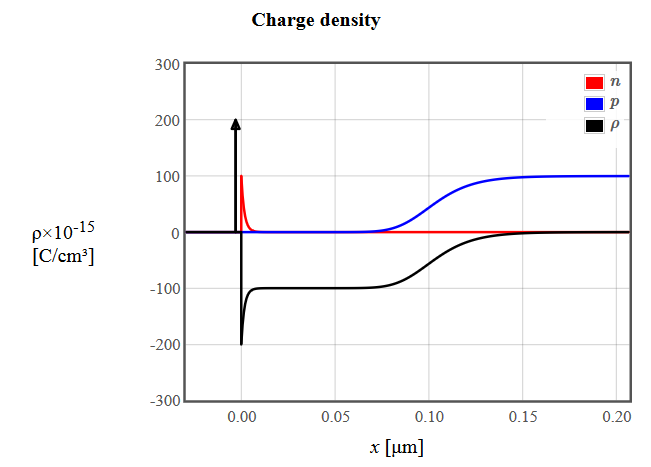
Problem 2
The charge density of a MOS capacitor is shown below.

(a) Is the semiconductor n-type or p-type?
(b) Draw the electric field that corresponds to this charge density. The electric field is positive if it points in the positive $x$-direction.
(c) Draw the band diagram that goes with this charge density.
(d) Is this MOS capacitor in accumulation, depletion, or inversion?
Problem 3
(a) You are given a silicon n-channel JFET. How can you experimentally determine the doping concentration in the channel?
(b) Plot the source-drain resistance of the JFET as a function of temperature. Assume no voltage is applied to the gate.
(c) The JFET is biased in saturation and at a certain drain voltage undergoes breakdown. How could you change JFET to increase the breakdown voltage?
(d) Where does diffusion occur in a JFET?
Problem 4
Consider a green light emitting diode.
(a) Draw the electron dispersion relation $E$ vs. $k$. Indicate the value of the bandgap $E_g$.
(b) Draw a cross section of a wafer with an LED. Include the contacts in the drawing.
(c) Describe the problems associated with coupling light out of the diode.
(d) What would you have to do to turn this LED into a laser diode?
Quantity | Symbol | Value | Units | |
| electron charge | e | 1.60217733 × 10-19 | C | |
| speed of light | c | 2.99792458 × 108 | m/s | |
| Planck's constant | h | 6.6260755 × 10-34 | J s | |
| reduced Planck's constant | $\hbar$ | 1.05457266 × 10-34 | J s | |
| Boltzmann's constant | kB | 1.380658 × 10-23 | J/K | |
| electron mass | me | 9.1093897 × 10-31 | kg | |
| Stefan-Boltzmann constant | σ | 5.67051 × 10-8 | W m-2 K-4 | |
| Bohr radius | a0 | 0.529177249 × 10-10 | m | |
| atomic mass constant | mu | 1.6605402 × 10-27 | kg | |
| permeability of vacuum | μ0 | 4π × 10-7 | N A-2 | |
| permittivity of vacuum | ε0 | 8.854187817 × 10-12 | F m-1 | |
| Avogado's constant | NA | 6.0221367 × 1023 | mol-1 |